How to effectively solve Motion in One dimension Problems

Motion in one dimension is one of the important chapters in Mechanics. This post is given to give the feel of the whole chapters plus the type of questions and how to solve motion in one dimension problem. This is quite beneficial for anybody studying One-dimensional Motion.

Description:
One dimensional Motion is the study of the motion along a straight line. The complete Study material has been provided at the below link
motion in one dimension

Most Important Points to remember about One dimension Motion
1. Distance and displacement are not the same things. Distance is scalar while displacement is a vector quantity. Distance is never zero in a round trip while the displacement will be zero. Example When a person moves in a circle and return to its normal position, its displacement is zero while the distance traveled is the circumference of the circle
2. Speed is calculated over distance while velocity is over displacement. So Average speed in a round trip will never be zero while average velocity will be zero
3. The magnitude of Instantaneous velocity is equal to the instantaneous speed
4. When a particle moves with constant velocity, its average velocity, its instantaneous velocity, and its speed are all equal
5. Acceleration is defined as the change in velocity per unit time. A body moving with constant speed but with varying directions will have acceleration as the velocity is changing.

Most Effective way to solve a motion in straight line problems

1. First, visualize the question
2. Take starting point as the origin and take one direction as positive and others as negative. This is required as we will be dealing with Vector quantities
3. Write down what is given in the question and what is required
4. If it is uniform motion, then you can utilize the One-dimensional motion equation. if the motion is varying and the relation is given for that motion. Then we can utilize a one-dimensional motion derivative equation to find out the solutions
5. Calculate as require

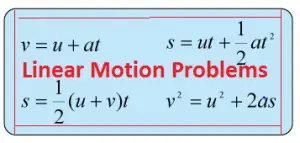
Formula’s of linear motion problems are
$v=u+at$
$s=ut+  \frac {1}{2}at^2$
$v^2=u^2+2as$
$x=\int v dt $
$v=\int a dt $
$v= \frac {dx}{dt}$
$a=\frac {dv}{dt}= \frac {d^2x}{dt^2}$
$a=v \frac {dv}{dx}$

Example -1
A bus starts at Station A from rest with a uniform acceleration of 2m/sec². The bus moves along a straight line
1. Find the distance moved by bus in 10 sec?
2. At what time, its velocity becomes 20 m/sec?
3 How much time it will take to cover a distance of 1.6 km

Solution:
Now first step to attempt such a question is to visualize the whole process. Here the bus is moving along a straight line and with uniform acceleration
Now what we have
Initial velocity=0
Acceleration=2m/sec²

Now since it is uniform motion we can use given motion formula’s in use
$v=u+at$
$s=ut+  \frac {1}{2}at^2$
$v^2=u^2+2as$

(1) distance (s)=?
time(t)=10 sec
So here the most suitable equation is
s=ut+(1/2)at²
Substituting given values
s=(0)10 +(1/2)2(10)²=100 m

(2) velocity(v)=20 m/s
time(t)=?
So here the most suitable equation is
v=u+at
20=0+2t
or t=10 sec

(3) distance(s)=1.6 km=1600 m
t=?
So here the most suitable equation is
s=ut+(1/2)at²
1600=(1/2)(2)t²
or t=40 sec

Example -2
An object is moving along a straight line. The motion of that object is described by
$x=at+bt^2+ct^3$
where a,b,c are constants and x is in meters and t is in sec.
1. Find the displacement at t=1 sec
2. Find the velocity at t=0 and t=1 sec
3. Find the acceleration at t=0 and t=1 sec

Solution:
Now first step to attempt such a question is to visualize the whole process. Here the object is moving along a straight line and its motion is described by the given equation
Now we
$x=at+bt^2+ct^3$
Now since its motion is described by the given equation,following formula will be useful in determining the values
$x=\int v dt $
$v=\int a dt $
$v= \frac {dx}{dt}$
$a=\frac {dv}{dt}= \frac {d^2x}{dt^2}$
$a=v \frac {dv}{dx}$

(1) x=? t=1sec

Here by substituting t=1 in given equation we get the answer
x=a(1)+b(1)²+c(1)³
x=a+b+c m

(2) v=? t=0,v=? t=1
Here we are having the displacement equation,so first we need to find out the velocity equation
So here the most suitable formula is
v=(dx/dt)
or v=d(at+bt²+ct³)/dt
or v=a+2bt+3ct²
Substituting t=0 we get
v=a m/s
Substituting t=1 we get
v=a+2b+3c m/s

(3) a=? t=0,a=? t=1
Now we are having the velocity equation, we need to first find the acceleration equation.
So here the most suitable formula is
a=(dv/dt)
or a=d(a+2bt+3ct²)/dt
a=2b+6ct
Substituting t=0 we get
a=2b m/s²
Substituting t=1 we get
a=2b+6c m/s²

Some other  good questions for your Assignments

(a) The displacement of the body x(in meters) varies with time t (in sec) as
x=(-2/3)t² +16t+2
find following
a. what is the velocity at t=0,t=1
b. what is the acceleration at t=0
c. what is the displacement at t=0
d .what will the displacement be when it comes to rest
e . How much time it takes to come to rest.

(b) A man runs at a speed at 4 m/s to overtake a standing bus. When he is 6 m behind the door at t=0, the bus moves forward and continues with a constant acceleration of 1.2 m/s²
find the following
a. how long does it take for the man to gain the door
b if in the beginning, he is 10m behind the door, will be running at the same speed ever catch up the bus?

Some Videos on how to solve motion in one dimension problem

Important Graph in One-dimensional Motion

A) Position Time Graph

This is a  plot of the Position and time of an object moving along the straight line. We can find out velocity, speed, distance, and displacement.  We can determine the acceleration sign from it but cannot determine the value of it

i) Velocity is given by the Slope of the Position time Graph. Velocity is positive if the slope is positive  and Velocity is negative if the slope is negative

ii) The numerical value of the Slope without sign gives the speed of the object

iii) Displacement can be calculated using position at the time interval

iv) Displacement-time graph is the same as Position Time graph. Distance time can be drawn from the position-time graph by taking the mirror of the image of the position-time graph from the point zero velocity onwards

iv) The slope of the chord drawn to displacement time or distance-time graph gives the average velocity or average speed  over the time interval to which the chords correspond to

v) If the graph is concave up, acceleration is + positive and If the graph is concave down, acceleration is -ve

B) Velocity Time graph

i) The area under the velocity-time curve provides the displacement

ii) The slope of the curve provides the acceleration

C) Acceleration -time Curve

i) The area under the curve provides the change in velocity

Hope you like this detailed post on how to solve motion in one dimension problem and it helps you in your studies

Related Articles
how to study physics problems
Solving physics problems
Describing Motion and Rest
kinematics conceptual questions and answers
Acceleration formula