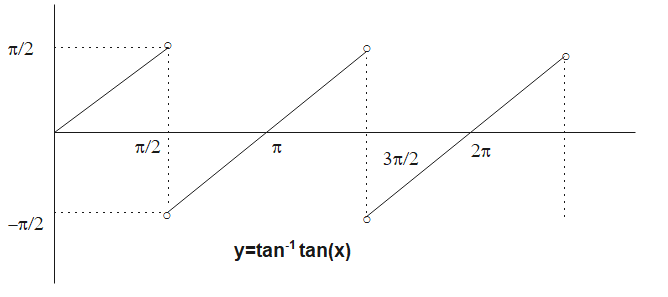
Here in this post ,we will check out how to draw the graph of tan inverse tan x i.e
$f(x) = tan^{-1} tan (x)$
As $tan (\pi +x ) = tan x$
This is a periodic function with period $\pi$
Also x cannot take values like $\frac {2n+1}{2} \pi$ as undefined
We know by definition
$f(x) = tan^{-1} tan (x) = x $ if $x \in (-\pi/2, \pi/2)$
Lets check out the other values
if $x \in (\pi/2, \pi]$
$\pi/2 < x \leq \pi $
Subtracting $\pi$ on all
$ \pi/2 – \pi< x – \pi \leq \pi -\pi $
$-\pi/2 < x- \pi \leq <0$
Also $tan (\pi -x) = -tan x$
or $ – tan( x – \pi) = -tan x$
or $tan( x – \pi) =tan x$
So $f(x) = tan^{-1} tan (x) = tan^{-1} tan (x – \pi)= x – \pi$
if $x \in (\pi, 3\pi/2)$
$\pi/< x < 3\pi/2 $
$ -3 \pi/2 < -x < -\pi$
Adding $\pi$
$- \pi/2 < \pi -x < 0$
or
$0 < x- \pi < \pi/2 <$
So $f(x) = tan^{-1} tan (x) = tan^{-1} tan (x – \pi)= x – \pi$
if $x \in (3\pi/2, 2\pi]$
$ 3 \pi/2 < x \leq 2\pi$
subtracting $2 \pi$ on all
$-\pi/2 \leq x – 2\pi < 0 $
Also $tan (2\pi -x) = -tan x$
or $ – tan( x -2 \pi) = -tan x$
or $tan( x -2 \pi) =tan x$
So $f(x) = tan^{-1} tan (x) = tan^{-1} tan (x – 2\pi)= x – 2\pi$
Therefore the graph will be