We often need to convert inverse of cos to either inverse of sin, tan, sec, cosec , cot .In this post we will see how we can do it easily
Inverse of cos to inverse of sin
Case 1
$cos^{-1} x$ and x > 0
Now we can write as
$\theta=cos^{-1} x$
$cos \theta =x$
Now we know that here $\theta \in [0,\pi/2]$, so it is an acute angle
Now it can be written as
$cos \theta =\frac {x}{1} = \frac {base}{hyp}$
In Right angle triangle
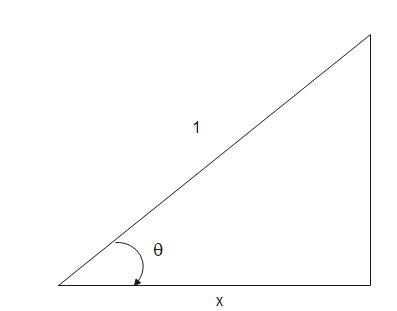
Now then perp becomes
$\text{perp} = \sqrt { 1 -x^2}$
So , $sin \theta = \frac {perp}{hyp} = \sqrt { 1 -x^2}$
or
$\theta= sin^{-1} \sqrt { 1 -x^2}$
or $cos^{-1} x = sin^{-1} \sqrt { 1 -x^2}$
Case II
$cos^{-1} x$ and x < 0
So value of the function will be in the range $[\pi/2 ,\pi]$
Now we know from the property that
$cos^{-1} (-x)= \pi – cos^{-1} (x)$
This can be written as
$cos^{-1} x = \pi – cos^{-1} |x| = \pi – sin^{-1} \sqrt { 1 -|x|^2}= \pi – sin^{-1} \sqrt { 1 -x^2}$
This makes sense also as Range of the cos and sin function differ. We can convert with out worrying about the sign in $[0, \pi/2]$ as it is common
Thus ,we have different formula depending on the values of x
Inverse of cos to inverse of tan
Case 1
$cos^{-1} x$ and x > 0
from the above, we can write that
$tan \theta = \frac {perp}{base} = \frac {\sqrt {1-x^2}}{x}$
or
$cos^{-1} x = tan^{-1} \frac {\sqrt {1-x^2}}{x}$
Case II
$cos^{-1} x$ and x < 0
Now we know from the property that
$cos^{-1} (-x)= \pi – cos^{-1} (x)$
This can be written as
$cos^{-1} x = \pi – cos^{-1} |x| =\pi – tan^{-1} \frac {\sqrt { 1 -x^2}}{|x|}=\pi + tan^{-1} \frac {\sqrt { 1 -x^2}}{x}$
This makes sense also as Range of the cos and tan function differ. We can convert with out worrying about the sign in $[0, \pi/2]$ as it is common
Thus ,we have different formula depending on the values of x
Inverse of cos to inverse of sec
This we already know from the property
$cos^{-1} x = sec^{-1} \frac {1}{x}$
for all values of x [-1,1]
Inverse of cos to inverse of cosec
Case 1
$cos^{-1} x$ and x > 0
from the above, we can write that
$cosec \theta = \frac {1}{\sqrt {1-x^2}}$
or
$cos^{-1} x = cosec^{-1} \frac {1}{\sqrt {1-x^2}}$
Case II
$cos^{-1} x$ and x < 0
So value of the function will be in the range $[\pi/2 ,\pi]$
Now we know from the property that
$cos^{-1} (-x)= \pi – cos^{-1} (x)$
This can be written as
$cos^{-1} x = \pi – cos^{-1} |x| = \pi – cosec^{-1} \frac {1}}{\sqrt { 1 -x^2}}$
This makes sense also as Range of the cos and sin function differ. We can convert with out worrying about the sign in $[0, \pi/2] as it is common
Thus ,we have different formula depending on the values of x
Inverse of cos to inverse of cot
Case 1
$cos^{-1} x$ and x > 0
from the above, we can write that
$cot \theta = \frac {x}{\sqrt {1-x^2}}$
or
$cos^{-1} x = cot^{-1} \frac {x}{\sqrt {1-x^2}}$
Case II
$cos^{-1} x$ and x < 0
So value of the function will be in the range $[\pi/2 ,\pi]$
Now we know from the property that
$cos^{-1} (-x)= \pi – cos^{-1} (x)$
This can be written as
$cos^{-1} x = \pi – cos^{-1} |x| = \pi – cot^{-1} \frac {|x|}}{\sqrt { 1 -x^2}}=cot^{-1} \frac {x}}{\sqrt { 1 -x^2}}$
So we have same formula for any values of x
$cos^{-1} x = cot^{-1} \frac {x}{\sqrt {1-x^2}}$