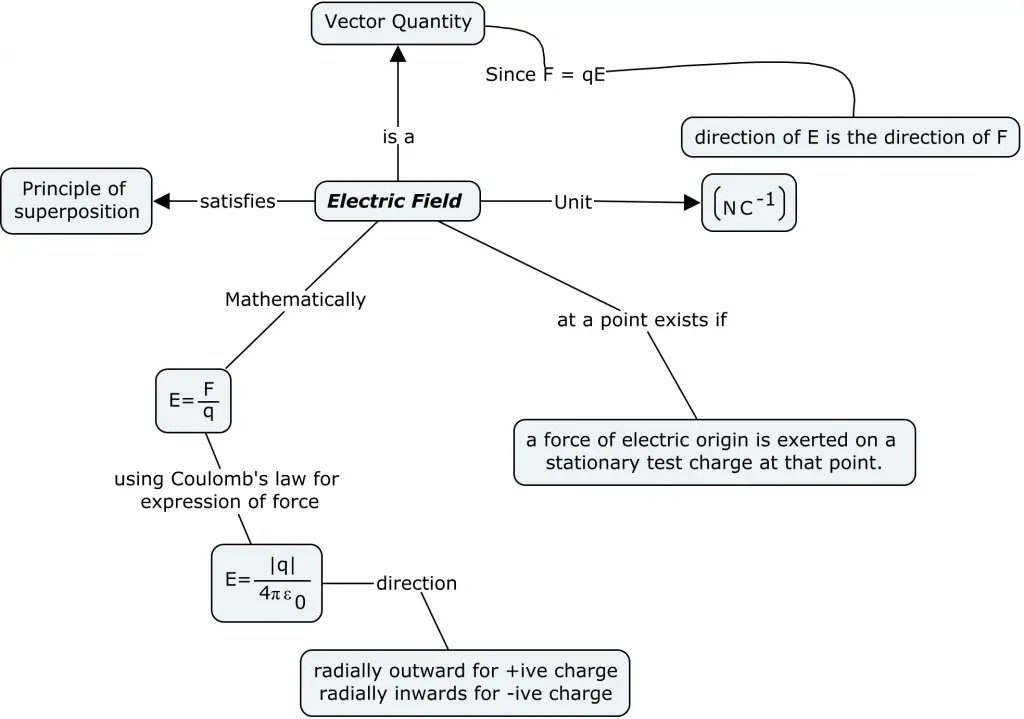
Since the car is turning on a curve, The car will be accelerating as direction of the car changes even the velocity is constant. This acceleration is centripetal acceleration And centripetal force will be providing it,
There are three cases with turning
1) Unbanked curve.
2) Banked curve with no friction
3) Banked curve with friction
Case -I Unbanked curve
In this particular case, The centripetal force is provided by the friction between tyres and the road. The friction acts inwards and provide the necessary force. If you draw the free body diagram of the car, You will notice , The vertical force Weight and Reaction balanced each other and frictional force is the net force acting on the car.
$\frac{mv^{2}}{r}=\mu mg$
$v=\sqrt{\mu rg}$
Some important points
1) Since frictional force is limiting,you cannot turn on high speed and you will be thrown outward due to inertia
2) You cannot turn the car on the ice road as friction will be absent
3) The max speed at which turning can happen depends on the friction coefficient and radius of the turn . It does not depend on the mass of the car
Case -II Banked curve with no friction
In first case, we understood that If a car is on a level (unbanked) surface, the forces acting on the car are its weight, mg, pulling the car downward, and the normal force, N, due to the road, which pushes the car upward. Both of these forces act in the vertical direction and have no horizontal component. And frictional force provide the necessary centripetal force for the turn and If there is no friction, there is no force that can supply the centripetal force required to make the car move in a circular path – there is no way that the car can turn.
Since in many cases friction could be less,the road are banked in order for the successful turning.The below paragraph explain it
if the car is on a banked turn, the normal force (which is always perpendicular to the road’s surface) is no longer vertical. The normal force now has a horizontal component, and this component can act as the centripetal force on the car! The car will have to move with just the right speed so that it needs a centripetal force equal to this available force, but it could be done. Given just the right speed, a car could safely negotiate a banked curve even if the road is covered with perfectly smooth ice!
The formula for the right speed is
$v=\sqrt{rgtan\theta }$
Some important points
1)With this approach ,if the friction is absent, the car can turn with the right speed only ,otherwise it will skid or slip
2) Since the speed does not depend on the mass, So as long the speed is same, truck and car will turn successfully
Case -III Banked curve with friction
In last case, we studied about the banked curved and we understood that if there is no friction, the object can turn with right speed on the banked curve.So what if the car move with higher speed than the right speed ,Frictional force required will be force and car will skid upward. Now if the friction is present , the frictional force will act inwards and provide the necessary centripetal force.
Similarly if the car is turning with speed less than right speed on the banked curve, it will try to skid inwards and frictional force will acts outward and the car can turn successfully
Related Articles
Force of friction equation
Atwood machine
fictitious force examples