Trigonometric equations are equations that involve trigonometric functions like sine, cosine, tangent, etc. The principal solutions of a trigonometric equation are the solutions that lie within a single period of the function i.e.. 0 to $2\pi$
Principal Solution: The solution in the range $0 \le x \le 2\pi$
How to find the Principal Solutions
We can easily find the Principal solutions using below method
(1)Now we know that from ” ALL SILVER TEA CUPS”
Sin is positive in First and second and it is negative in 3rd and 4th Quadrant
Cos is positive in First and fourth and it is negative in 2 and 4th Quadrant
tan is positive in first and third and its negative in 2 and 3 quadrant
Hence if the sin is positive, the principal solution will in first and second quadrant and if it is negative , principal solutions will be third and fourth quadrant
if the cos is positive, the principal solution will in first and fourth quadrant and if it is negative , principal solutions will be second and third quadrant
if the tan is positive, the principal solution will in first and third quadrant and if it is negative , principal solutions will be second and fourth quadrant
(2)Now we are also aware of these identities
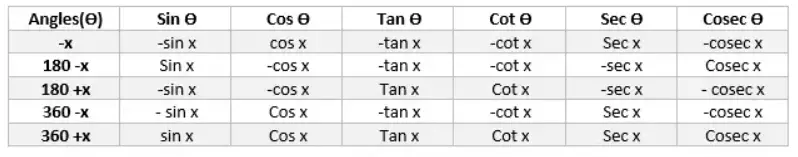
This table is very easy to remember, as each correspond to same function. The sign is decided by the corresponding sign of the trigonometric function of the angle in the quadrant
(3) Now we need to Find the value of the angle using the positive value.
(4) Now using the above identities and sign knowledge , we can find the principle values
Example 1
$\sin x = \frac {1}{2}$
Here it is positive, so the principal values will be in first and second quadrant
we can write as
$\sin x = \sin \frac {\pi}{6}$
So one principle value will be $\frac {\pi}{6}$
Now we know that other will be in second quadrant, so from the table taking the second quadrant identity
$sin (\pi -x) = sin x$
$\sin (\pi – \frac {\pi}{6}) = \sin\frac {\pi}{6}$
or
$\sin \frac {5 \pi}{6} = \sin\frac {\pi}{6}$
Hence the second value is $\frac {5\pi}{6}$
So, principal values are $\frac {\pi}{6}$ and $\frac {5\pi}{6}$
Example 2
$\sin x = -\frac {1}{2}$
Here it is negative, so the principal values will be in third and fourth quadrant
we can write as
$\sin x = -\sin \frac {\pi}{6}$
Now we know that first will be in third quadrant, so from the table taking the third quadrant identity
$sin (\pi +x) = -sin x$
$sin (\pi + \frac {\pi}{6}) = -\sin \frac {\pi}{6}$
$sin \frac {7\pi}{6}= -\sin \frac {\pi}{6}$
So first principal value is $\frac {7\pi}{6}$
Now we know that second will be in fourth quadrant, so from the table taking the fourth quadrant identity
$sin (2\pi – x) = -sin x$
$sin (2\pi – \frac {\pi}{6}) = -\sin \frac {\pi}{6}$
$sin \frac {11\pi}{6}= -\sin \frac {\pi}{6}$
So first principal value is $\frac {11\pi}{6}$
So, principal values are $\frac {7\pi}{6}$ and $\frac {11\pi}{6}$
Example 3
$\cos x = \frac {1}{2}$
Here it is positive, so the principal values will be in first and fourth quadrant
we can write as
$\cos x = \cos \frac {\pi}{3}$
So one principle value will be $\frac {\pi}{3}$
Now we know that other will be in fourth quadrant, so from the table taking the fourth quadrant identity
$cos (2\pi -x) = cos x$
$\cos (2\pi – \frac {\pi}{3}) = \cos \frac {\pi}{3}$
or
$\cos \frac {5 \pi}{3} = \cos \frac {\pi}{3}$
Hence the second value is $\frac {5\pi}{3}$
So, principal values are $\frac {\pi}{3}$ and $\frac {5\pi}{3}$
Example 4
$\cos x = -\frac {1}{2}$
Here it is negative, so the principal values will be in second and third quadrant
we can write as
$\cos x = -\cos \frac {\pi}{3}$
Now we know that first will be in second quadrant, so from the table taking the second quadrant identity
$cos (\pi -x) = -cos x$
$\cos (\pi – \frac {\pi}{3}) =- \cos \frac {\pi}{3}$
or
$\cos \frac {2 \pi}{3} = -\cos \frac {\pi}{3}$
Hence the First value is $\frac {2\pi}{3}$
Now we know that second will be in third quadrant, so from the table taking the third quadrant identity
$cos (\pi +x) = -cos x$
$\cos (\pi + \frac {\pi}{3}) =- \cos \frac {\pi}{3}$
or
$\cos \frac {4 \pi}{3} = -\cos \frac {\pi}{3}$
Hence the second value is $\frac {4\pi}{3}$
So, principal values are $\frac {2\pi}{3}$ and $\frac {4\pi}{3}$
Example 5
$\tan x = 1$
Here it is positive, so the principal values will be in first and third quadrant
we can write as
$\tan x = \tan \frac {\pi}{4}$
So one principle value will be $\frac {\pi}{4}$
Now we know that other will be in third quadrant, so from the table taking the third quadrant identity
$tan (\pi +x) = tan x$
$\tan (\pi + \frac {\pi}{4}) = \tan \frac {\pi}{4}$
or
$\tan \frac {5 \pi}{4} = \tan \frac {\pi}{4}$
Hence the second value is $\frac {5\pi}{4}$
So, principal values are $\frac {\pi}{4}$ and $\frac {5\pi}{4}$
Example 6
$\tan x == 1$
Here it is negative, so the principal values will be in second and fourth quadrant
we can write as
$\tan x = -\tan \frac {\pi}{4}$
Now we know that first will be in second quadrant, so from the table taking the second quadrant identity
$tan (\pi -x) = -tan x$
$\tan (\pi – \frac {\pi}{4}) = -\tan \frac {\pi}{4}$
or
$\tan \frac {3 \pi}{4} =- \tan \frac {\pi}{4}$
Hence the first value is $\frac {3\pi}{4}$
Now we know that second will be in fourth quadrant, so from the table taking the fourth quadrant identity
$tan (2\pi -x) = -tan x$
$\tan (2\pi – \frac {\pi}{4}) = -\tan \frac {\pi}{4}$
or
$\tan \frac {7 \pi}{4} =- \tan \frac {\pi}{4}$
Hence the first value is $\frac {7\pi}{4}$
So, principal values are $\frac {3\pi}{4}$ and $\frac {7\pi}{4}$