How to Find values of Sin 18, cos 18, tan 18, sin 36,cos 36, Sin 54, cos 72

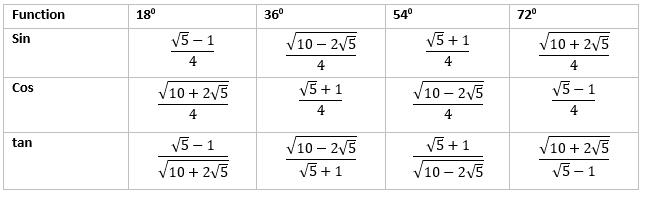
We will be checking how to find the values of Sin 18, cos 18, tan 18, sin 36,cos 36 degrees with the normal known values of the trigonometric ratios i.e with out the use the calculator

Value of sin 18 degrees

Let   $A = 18$

Now, we need to convert into known common values,so

$5A = 90$

$2A + 3A = 90$

$2A = 90 – 3A$

Taking Sin on both the sides, we get

$sin (2A) = sin (90 – 3A)$

As we know that sin (90 – 3A) =cos (3A),So

$sin (2A)  =cos (3A)$

Now we need to convert them into single angle functions

$sin 2A = 2 Sin (A) Cos(A)$

$cos (3A) = 4 cos^3 (A) – 3 cos (A)$

Substituting these values

$2 Sin (A) Cos(A)= 4 cos^3 (A) – 3 cos (A)$

Rearranging them , we get

$4 cos^3 (A) – 3 cos (A) – 2 Sin (A) Cos(A)=0$

$cos (A) ( 4 Cos^2 A – 2 sin (A) -3)=0$

Now we know that $cos A \ne 0 $,So

$  4 Cos^2 (A) – 2 sin (A) -3=0$

$4( 1-sin^2 (A) )- 2 sin (A) -3=0$

$4 sin^2 (A) + 2 Sin(A)  -1=0$

This is a quadratic equation , Solving using Quadratic Formula

$sin (A) = \frac {-2 \pm \sqrt {4 + 16}} {8}$

$ =\frac {-2 \pm \sqrt {20}} {8}$

$=\frac {-2 \pm  2\sqrt {5}} {8}$

$ =\frac {-1 \pm  \sqrt {5}} {4}$

As we know that sin 18 > 0,

So $sin (A) = \frac {-1 +  \sqrt {5}} {4}$

Hence $sin (18^0)= \frac {-1 +  \sqrt {5}} {4}$

Value of cos 18 degrees

We know from trigonometric identity that

$sin^2 A +  cos^2 A = 1$

or

$sin^2 (18) +  cos^2 (18) = 1$

$cos^2 (18) = 1 – sin^2 (18)$

$cos ^2 (18) = 1 – \left ( \frac {-1 +  \sqrt {5}} {4} \right ) ^2 $

$cos ^2 (18) = 1 – \frac { 6 – 2 \sqrt {5}}{16} $

$cos^2 (18) = \frac { 10 + 2  \sqrt {5}}{16}$

$cos (18)= \frac {\sqrt {10 + 2  \sqrt {5}}}{4}$

we ignore the negative value as cos 18 > 0

Value of tan 18 degrees

We know that

$tan (18) = \frac {sin (18) }{cos (18)}$

Substituting the values we get from above

$tan (18)  = \frac { -1 + \sqrt {5}}{\sqrt {10 + 2  \sqrt {5}}}$

Value of sin 36 degrees

Now  as

$sin (2A) = 2 sin (A) cos (A) $

$ sin (36) = 2 sin (18) cos (18) $

$sin (36) = 2 \times \frac {-1 +  \sqrt {5}} {4} \times \frac {\sqrt {10 + 2  \sqrt {5}}}{4}$

$sin (36) = \frac { \sqrt { (-1 +  \sqrt {5})^2(10 + 2  \sqrt {5})}}{8}$

$sin (36) = \frac {\sqrt {10 -2 \sqrt {5}}}{4}$

Value of cos 36 degrees

We know from trigonometric identity that

$sin^2 A +  cos^2 A = 1$

or

$sin^2 (36) +  cos^2 (36) = 1$

$cos^2 (36) = 1 – sin^2 (36)$

$cos ^2 (36) = 1 – \left ( \frac {\sqrt {10 -2 \sqrt {5}}}{4} \right ) ^2 $

$cos ^2 (36) = 1 – \frac { 10 – 2 \sqrt {5}}{16} $

$cos^2 (36) = \frac { 6 + 2  \sqrt {5}}{16} = \frac {(1 + \sqrt {5})^2}{4^2}$

$cos (36)= \frac {1 + \sqrt {5}}{4}$

we ignore the negative value as cos 36 > 0

Value of Sin 54 degrees

Now

$ sin (54) = sin ( 90 -36) =cos 36 =  \frac {1 + \sqrt {5}}{4}$

Value of Cos 54 degrees

$ cos (54) = cos ( 90 -36) =sin  36 = \frac {\sqrt {10 -2 \sqrt {5}}}{4}$

Value of Sin 72 degrees

$ sin (72) = sin ( 90 -18) =cos 18 = \frac {\sqrt {10 + 2  \sqrt {5}}}{4}$

Value of cos 72 degrees

$ cos (72) = cos ( 90 -18) =sin 18 = \frac {-1 +  \sqrt {5}} {4}$

Summary

Also Reads
Trigonometric table from 0 to 360
Sin 15 degrees
Trigonometry Formulas for class 11 (PDF download)
Differentiation formulas
https://en.wikipedia.org/wiki/Trigonometry