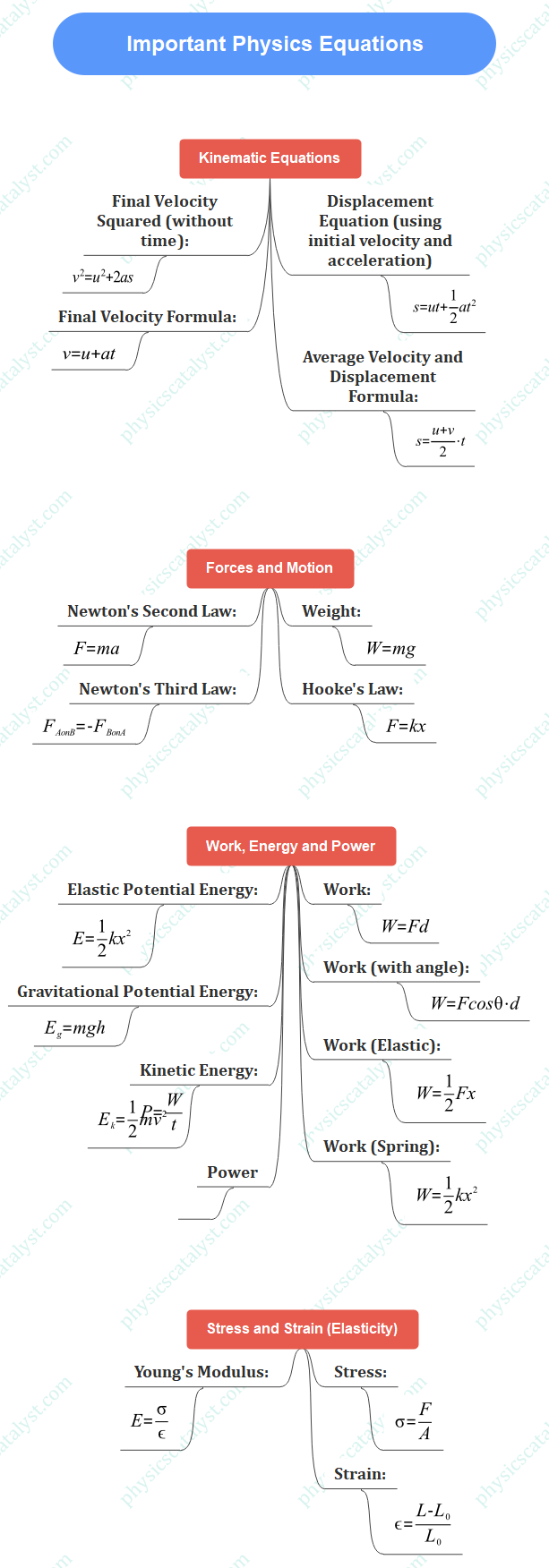
Essential mechanics Equations for Physics Students
Master the essential mechanics equations with this comprehensive guide! From kinematics to work and energy, stress and strain, and more—this post explains key concepts with easy-to-understand equations. Download the full PDF and explore a mind map for effective memorization and quick revision!
Kinematic Equations (Constant Acceleration)
These equations describe the motion of an object under constant acceleration. They relate the displacement, initial velocity, final velocity, acceleration, and time taken.
Displacement Equation (using initial velocity and acceleration):
$$s = ut + \frac{1}{2}at^2$$
Explanation:
- $s$= displacement (how far the object travels)
- $u$= initial velocity (velocity at the start)
- $t$= time (how long the object is in motion)
- $a$= acceleration (the rate at which the velocity is changing)
This equation gives the total displacement of an object when you know its initial velocity, acceleration, and the time of travel.
Average Velocity and Displacement Formula:
$$s = \frac{u + v}{2} \cdot t$$
Explanation:
- $s$= displacement
- $u$= initial velocity
- $v$= final velocity
- $t$= time
This equation calculates displacement by finding the average of the initial and final velocity, then multiplying it by the time. It is often used when acceleration isn’t given but velocities are known.
Final Velocity Formula:
$$v = u + at$$
Explanation:
- $v$= final velocity
- $u$= initial velocity
- $a$= acceleration
- $t$= time This equation gives the final velocity of an object after it has been accelerating at a constant rate for a given period of time.
Final Velocity Squared (without time):
$$v^2 = u^2 + 2as$$
Explanation:
- $v$= final velocity
- $u$= initial velocity
- $a$= acceleration
- $s$= displacement This equation calculates the final velocity squared without needing the time. It uses the initial velocity, acceleration, and displacement to determine how fast the object will be moving at the end of its motion.
Summary:
These kinematic equations are useful for solving problems involving linear motion with constant acceleration. Depending on the known quantities (initial/final velocity, acceleration, displacement, or time), different equations can be applied to solve for unknowns in the motion of an object.
Forces and Motion – Explanation of Equations
Newton’s Second Law:
$$F = ma$$
Explanation:
- $F$= force (measured in newtons, N)
- $m$= mass of the object (measured in kilograms, kg)
- $a$= acceleration (measured in meters per second squared, $m/s^2$)
Newton’s Second Law states that the force acting on an object is equal to the mass of the object multiplied by its acceleration. This equation shows that as an object’s mass increases, so does the force required to move it. Similarly, the faster you want an object to speed up or accelerate, the more force is required to push it.
It explains how the motion of an object changes when a force is applied to it. For example, pushing a heavy cart (larger $m$) requires more force ($F$) to accelerate it to the same speed as a lighter cart.
Newton’s Third Law:
$$F_{\text{A on B}} = – F_{\text{B on A}}$$
Explanation:
- $F_{\text{A on B}}$= the force that object A exerts on object B
- $F_{\text{B on A}}$= the force that object B exerts on object A
- The negative sign indicates that the forces are equal in magnitude but opposite in direction.
Newton’s Third Law states that for every action, there is an equal and opposite reaction. In other words, when one object exerts a force on another, the second object exerts an equal force back on the first object, but in the opposite direction.
For example, when you push against a wall, the wall pushes back against you with the same amount of force. It’s like trying to push a door that someone else is pushing from the other side with the same strength.
Weight:
$$W = mg$$
Explanation:
- $W$= weight (measured in newtons, N)
- $m$= mass (measured in kilograms, kg)
- $g$= acceleration due to gravity (typically $9.8 \, m/s^2$on Earth)
Weight is the force exerted by gravity on an object. This equation shows that an object’s weight is proportional to its mass and the gravitational field strength. On Earth, this acceleration due to gravity is approximately $9.8 \, m/s^2$.
Weight is a vector quantity, meaning it has both magnitude and direction. On Earth, the direction of weight is downward due to the Earth’s gravitational pull.
For example, an object with a mass of $10 \, \text{kg}$will have a weight of $W = 10 \times 9.8 = 98 \, \text{N}$.
Hooke’s Law:
$$F = kx$$
Explanation:
- $F$= restoring force (measured in newtons, N)
- $k$= spring constant (measured in newtons per meter, $N/m$), representing the stiffness of the spring
- $x$= displacement from the equilibrium position (measured in meters, m)
Hooke’s Law describes the force exerted by a spring when it is compressed or stretched. The force is proportional to the displacement $x$ and the spring constant $k$, which indicates how stiff the spring is. The greater the displacement or the stiffer the spring, the larger the restoring force.
For example, if you stretch a spring by $0.2 \, \text{m}$with a spring constant of $100 \, N/m$, the restoring force will be $F = 100 \times 0.2 = 20 \, N$.
Summary:
These equations form the foundation of understanding forces and motion:
- Newton’s Second Law quantifies the relationship between force, mass, and acceleration.
- Newton’s Third Law explains the mutual forces between interacting objects.
- Weight represents the gravitational force on an object due to its mass.
- Hooke’s Law describes the behavior of springs and other elastic objects when they are deformed.
These laws and formulas help describe and predict how objects move and interact in physical systems.
Work and Energy – Explanation of Equations
These equations describe the concepts of work and various forms of energy, including kinetic, potential, and elastic energies. Let’s go through them one by one.
Work:
$$W = Fd$$
Explanation:
- $W$= work (measured in joules, J)
- $F$= force applied (measured in newtons, N)
- $d$= displacement (measured in meters, m)
Work is the amount of energy transferred by a force acting over a distance. It is calculated by multiplying the force applied to an object by the displacement of the object in the direction of the force. If there is no displacement, no work is done, even if a force is applied.
For example, if you push a box with a force of 10 N over a distance of 2 meters, the work done is $W = 10 \times 2 = 20 \, \text{J}$.
Work (with Angle):
$$W = F \cos \theta \cdot d$$
Explanation:
- $W$= work (measured in joules, J)
- $F$= force applied (measured in newtons, N)
- $\theta$= angle between the force and the direction of displacement
- $d$= displacement (measured in meters, m)
This version of the work formula is used when the force is not applied in the same direction as the displacement. The term $\cos \theta$ accounts for the angle between the force and displacement directions. Only the component of the force in the direction of the displacement does work.
For example, if you pull a sled at an angle of $30^\circ$ with a force of 50 N for a distance of 5 meters, the work done is $W = 50 \cos 30^\circ \times 5 \approx 216.5 \, \text{J}$.
Work (Elastic):
$$W = \frac{1}{2}Fx$$
Explanation:
- $W$= work (measured in joules, J)
- $F$= force applied (measured in newtons, N)
- $x$= displacement (measured in meters, m)
Elastic work is the energy stored in an elastic object when it is stretched or compressed. Imagine stretching a spring. As you pull it further, the force required to keep it stretched increases. This means that the force isn’t constant throughout the stretching process. To calculate the total work done, we can find the average force, which is half of the maximum force ($\frac{1}{2}F$). This average force multiplied by the displacement gives us the elastic work.
For example, if a spring is stretched by 0.5 meters and the force required to stretch it is 20 N, the work done is $W = \frac{1}{2} \times 20 \times 0.5 = 5 \, \text{J}$.
Work (Spring):
$$W = \frac{1}{2}kx^2$$
Explanation:
- $W$= work (measured in joules, J)
- $k$= spring constant (measured in newtons per meter, N/m)
- $x$= displacement from the equilibrium position (measured in meters, m)
Work (Spring) calculates the work needed to stretch or compress a spring by a distance $x$. The equation uses the spring constant $k$, which measures how stiff the spring is, and the displacement $x$, the amount the spring is stretched or compressed.
For example, if a spring with a constant of $100 \, N/m$is stretched by 0.3 meters, the work done is $W = \frac{1}{2} \times 100 \times (0.3)^2 = 4.5 \, \text{J}$.
Kinetic Energy:
$$E_k = \frac{1}{2}mv^2$$
Explanation:
- $E_k$= kinetic energy (measured in joules, J)
- $m$= mass of the object (measured in kilograms, kg)
- $v$= velocity of the object (measured in meters per second, m/s)
Kinetic Energy is the energy an object has due to its motion. It depends on the object’s mass and the square of its velocity. The faster an object moves and the more massive it is, the greater its kinetic energy.
For example, if a car with a mass of $1500 \, \text{kg}$is moving at $20 \, \text{m/s}$, its kinetic energy is $E_k = \frac{1}{2} \times 1500 \times 20^2 = 300,000 \, \text{J}$.
Gravitational Potential Energy:
$$E_g = mgh$$
Explanation:
- $E_g$= gravitational potential energy (measured in joules, J)
- $m$= mass of the object (measured in kilograms, kg)
- $g$= acceleration due to gravity (approximately $9.8 \, m/s^2$on Earth)
- $h$= height above a reference point (measured in meters, m)
Gravitational Potential Energy is the energy stored in an object due to its position in a gravitational field. The higher the object is, the more potential energy it has. This energy is directly proportional to the object’s height and mass. For example, if you lift a book with a mass of $2 \, \text{kg}$to a shelf 1.5 meters high, its gravitational potential energy is $E_g = 2 \times 9.8 \times 1.5 = 29.4 \, \text{J}$.
Elastic Potential Energy:
$$E = \frac{1}{2}kx^2$$
Explanation:
- $E$= elastic potential energy (measured in joules, J)
- $k$= spring constant (measured in newtons per meter, N/m)
- $x$= displacement (measured in meters, m)
Elastic Potential Energy is the energy stored in an elastic object, such as a spring, when it is stretched or compressed. Like work done on a spring, this energy depends on the spring constant and the square of the displacement from equilibrium.
The equation for elastic potential energy is the same as the equation for work done on a spring, emphasizing the connection between these two concepts.
For example, if a spring with a spring constant of $200 \, N/m$ is compressed by 0.25 meters, the elastic potential energy stored in the spring is $E = \frac{1}{2} \times 200 \times (0.25)^2 = 6.25 \, \text{J}$.
Summary:
These equations describe the work done by forces and the various forms of energy in physical systems:
- Work quantifies energy transfer due to forces acting over a distance.
- Kinetic energy relates to an object’s motion, while potential energies (gravitational and elastic) describe stored energy based on position or deformation.
Stress and Strain (Elasticity) – Explanation of Equations
These equations describe the properties of materials when they undergo deformation due to applied forces. They relate to the elasticity of materials, quantifying the internal forces and deformations they experience.
Stress:
$$\sigma = \frac{F}{A}$$
Explanation:
- $\sigma$= stress (measured in pascals, Pa, or N/m²)
- $F$= force applied (measured in newtons, N)
- $A$= cross-sectional area of the material (measured in square meters, $m^2$)
Stress is a measure of the internal force exerted per unit area within a material. It is a response to external forces acting on the material and is directly proportional to the force and inversely proportional to the area over which the force is applied. This concept is used to assess how materials handle forces, such as tension or compression.
For example, if a force of 200 N is applied over an area of $0.02 \, m^2$, the stress is $\sigma = \frac{200}{0.02} = 10,000 \, \text{Pa}$.
Strain:
$$\epsilon = \frac{L – L_0}{L_0}$$
Explanation:
- $\epsilon$= strain (dimensionless, as it is a ratio)
- $L_0$= original length of the material (measured in meters, m)
- $L$= final length of the material after deformation (measured in meters, m)
Strain is the measure of deformation or elongation of a material due to an applied force. It is a dimensionless quantity because it is the ratio of the change in length to the original length. Strain quantifies how much a material stretches or compresses under stress.
For example, if a material’s original length is 1 meter and it elongates to 1.05 meters under stress, the strain is $\epsilon = \frac{1.05 – 1}{1} = 0.05$.
Young’s Modulus (Elastic Modulus):
$$E = \frac{\sigma}{\epsilon}$$
Explanation:
- $E$= Young’s modulus (measured in pascals, Pa)
- $\sigma$= stress (measured in pascals, Pa)
- $\epsilon$= strain (dimensionless)
Young’s Modulus is a measure of a material’s stiffness or elasticity. It relates stress to strain and describes how much a material will deform under a given load. A higher Young’s modulus indicates a stiffer material that will deform less when subjected to the same stress. For example, steel has a higher Young’s modulus than rubber, meaning that steel is much stiffer and deforms less than rubber under the same applied force.
In linear elastic materials, stress and strain are directly proportional. The slope of the stress-strain curve is equal to Young’s modulus, which quantifies the material’s stiffness or resistance to deformation.
Power – Explanation of Equation
Power is a measure of how fast work is done or how quickly energy is transferred. It is essential in physics for understanding how energy is used over time.
Power:
$$P = \frac{W}{t}$$
Explanation:
- $P$= power (measured in watts, W)
- $W$= work done (measured in joules, J)
- $t$= time taken (measured in seconds, s)
Power quantifies the rate at which work is done or energy is transferred. One watt is defined as one joule of energy transferred per second. The faster the work is done, the higher the power. For example, if 100 joules of work is done in 5 seconds, the power is $P = \frac{100}{5} = 20 \, \text{W}$.
Summary:
- Stress and strain describe how materials respond to forces and how much they deform.
- Young’s modulus gives a material’s stiffness or resistance to deformation.
- Power is a measure of how quickly work is done or energy is used over time.
Physics mechanics formula Mind Map
 Skip to content
Skip to content