Latest Articles

LCM and HCF questions for Class 6 Maths Playing With Numbers

In this page we have HCF and LCM questions for Class 6 Maths Playing With Numbers . Hope you like them and do not forget to like , social share and comment at the end of the page.

Question 1
Find the LCM of 12, 16, 24 and 36?

Answer

$12= 2 \times 2 \times 3$
$16 = 2 \times 2 \times 2 \times 2$
$24 = 2 \times 2 \times 2 \times 3$
$36= 2 \times 2 \times 3 \times 3$
LCM = $2 \times 2 \times 2 \times 2 \times 3 \times 3 =144$

Question 2
Find the HCF of 70, 105, 175

Answer

$70 = 2 \times 5 \times 7$
$105 = 3 \times 7 \times 5$
$175 = 5 \times 5 \times 7$
HCF =$5 \times 7 =35$

Question 3
What are twin primes? Give four examples

Answer

Two prime numbers whose difference is 2 are called twin primes.
For example:

3 and 5 are twin primes
5 and 7 are twin primes

Question 4
What is a prime triplet? Give an example.

Answer

A prime triplet is a set of three prime numbers of the form:

(p,p+2,p+6) or (p,p+4,p+6)

Some Examples are (5, 7, 11), (7, 11, 13), (11, 13, 17)

Question 5
Arrange the prime factors of 1350 in descending order.

Answer

$1350 = 2 \times 3 \times 3 \times 3 \times 5 \times 5$
Prime factors in descending order
5 > 3 >2

Question 6
Find the LCM of the following numbers:
a. 11 and 4
b. 125 and 5
c. 12 and 16
d. 8, 64

Answer

a. 44
b. 125
c. 48
d. 64

Question 7
Express 53 as the sum of three odd primes.

Answer

53 = 13 + 17 + 23

or 53 = 7 + 17 + 29

Question 8
In a morning walk, three persons step off together. Their steps measure 80 cm, 85 cm and 90 cm respectively. What is the minimum distance each should walk so that all can cover the same distance in complete steps?

Answer

We have to find the LCM of 80 cm, 85 cm and 90 cm
LCM =12240 cm

Question 9
Find the least number which when divided by 12, 16 ,24 and 36 leaves a remainder 7 in each case.

Answer

LCM of 12,16,24 and 36 =144
Least Number = 144 + 7 = 151

Question 10
Find the multiples of 11 which is greater than 56 but less than 79

Answer

Multiples of 11 = 11,22,33,44,55,66,77,88

So multiples greater than 56 but less than 79 are 66 and 77

Question 11
Find the HCF of the following numbers:
(a) 11, 44
(b) 35, 42
(c) 128, 60
(d) 81, 63
(e) 36, 180
(f) 1152, 1664
(g) 70, 115, 175
(h) 91, 112, 49
(i) 18, 54, 81
(j) 12, 45, 85

Answer

1.
a.
11= 11
44 =11 X 2 X 2
HCF =11
b.
35 = 5 x 7
42 = 7 x 3 x 2
HCF =7
c.
128= 2 x 2 x 2 x 2 x 2 x 2 x 2
60 = 2 x 2 x 3 x 5
HCF =4
d. 81 = 3 x 3 x3 x3
63 = 7 x 3 x 3
HCF =9
e.
36 =2 x 2 x3 x3
180 = 2 x 2 x 5 x 3 x 3
HCF = 12
f.
1152=2 x 2 x 2 x 2 x 2 x 2 x 2 x3 x3
1664=2 x 2 x 2 x 2 x 2 x 2 x 2 x 13
HCF= 128
g.
70 =2 x 5 x 3 x 3
115= 23 x 5
175 = 5 x 5 x7
HCF = 5
h.7
i. 9
j. 1

Question 12
Find the LCM of the following numbers:
(a) 4,7,12,84
(b) 25,15,36
(c) 24,36,40
(d) 27,36
(e) 4,8,18
(f) 2,3,4,5,6,7

Answer

a.
$4=2 \times 2$
$7= 7 \times 1$
$12 =2 \times 2 \times 3$
$84= 2 \times 2 \times 3 \times 7$
LCM =$2 \times 2 \times 3 \times 7 =84$

b.
$25 = 5 \times 5$
$15 = 5 \times 3$
$36 = 2 \times 2 \times 3 \times 3$
LCM =$2 \times 2 \times 3 \times 3 \times 5 \times 5 =900$

c.
$24=2 \times 2 \times 2 \times 3$
$36= 2 \times 2 \times 3 \times 3$
$40= 2 \times 2 \times 2 \times 5$
LCM =$2 \times 2 \times 2 \times 3 \times 3 \times 5= 360$

d.
$27 = 3 \times 3 \times 3$
$36=2 \times 2 \times 3 \times 3$
LCM =$2 \times 2 \times 3 \times 3 \times 3 = 108$

e. 72
f. 420

Question 13
You have 24 small bottles of lemonade and 36 large bottles. What is the largest number of equal-sized groups you can make if each group has the same number of small and large bottles?

Answer

24 small bottles and 36 large bottles
HCF of 24 and 36 = 12
Largest number of equal-sized groups = 12

Question 14
Sita has two ribbons, one of length 30 cm and the other of length 45 cm. She wants to cut them into smaller pieces of equal length without any ribbon left over. What is the greatest possible length of each piece?

Answer

30 cm and 45 cm ribbons
HCF of 30 and 45 = 15
Greatest possible length of each piece = 15 cm

Question 15
A bookshelf contains 84 science books and 108 mathematics books. If the books are to be arranged in shelves such that each shelf has the same number of books and only one type of book on each shelf, what is the maximum number of books that can be placed on each shelf?

Answer

84 science books and 108 mathematics books
HCF of 84 and 108 = 12
Maximum number of books on each shelf = 12

Question 16
Three tankers can contain 391 litres, 483 litres and 667 litres of diesel respectively. Find the maximum capacity of a container which can measure the diesel of the three containers exact number of time?

Answer

To solve this problem, we need to find the Highest Common Factor (HCF) of the three given quantities: 391 litres, 483 litres, and 667 litres. The HCF will give us the maximum capacity of a container that can measure the diesel in each of these tankers an exact number of times.
391 = 17 × 23
483 = 3 × 7 × 23
667 = 23 × 29
So the HCF is 23.

Question 17
In a school library there are 1080 books of Mathematics and 792 books of Science. Ms Shamita , the librarian of the school wants to store these books in shelves such that each shelf should have the same number of books of each subject. What should be the minimum number of each subject in each shelf?

Answer

Prime factorization of 1080: $2^3 \times 3^3 \times 5$
Prime factorization of 792: $2^3 \times 3^2 \times 11$
Therefore, the HCF is =8×9=72.
The minimum number of each subject that should be placed on each shelf is 72 books.

Question 18
Three lights blink at intervals of 4 seconds, 6 seconds, and 8 seconds, respectively. If they all blink together at 12:00 PM, when will they blink together again?

Answer

To find when they all blink together again, find the LCM of 4, 6, and 8.
Prime factorization of 4: 2 x 2
Prime factorization of 6: 2 x 3
Prime factorization of 8: 2 x 2 x 2
LCM = 24 seconds.
All lights will blink together again after 24 seconds.

Question 19
Six bells commence tolling together and toll at intervals of 2,4,6,8,10,12 minutes respectively in 24 hours , how many times do they toll together ?

Answer

Six bells commence tolling together and toll at intervals of 2,4,6,8,10,12 minutes respectively.
Find LCM of using prime factorization :
2=2
4=2 x 2
6 =2 x 3
8 =2 x 2 x2
10= 2 x 5
12= 2 x 2 x 3
Therefore LCM (2,4,6,8,10,12) = 120 min
After every 120 minutes = 2 hours , bells will toil together.
So required Number of times = 13

Question 20
Floor of a room measures 4.5 metres x 3 metres. Find the minimum number of complete square marble slabs of equal size required to cover the entire floor.

Answer

To find the minimum number of square slabs to cover the floor, we have to find the greatest size of each such slab. For this purpose, we have to find the HCF of 450 and 300.
(Since 4.5m = 450cm and 3m = 300cm)
Now HCF of 450 and 300 = 150
So the required size of the slab must be 150cm × 150cm.
Hence, the number of slabs required =Area of the floor/ Area of 1 Slab= 6

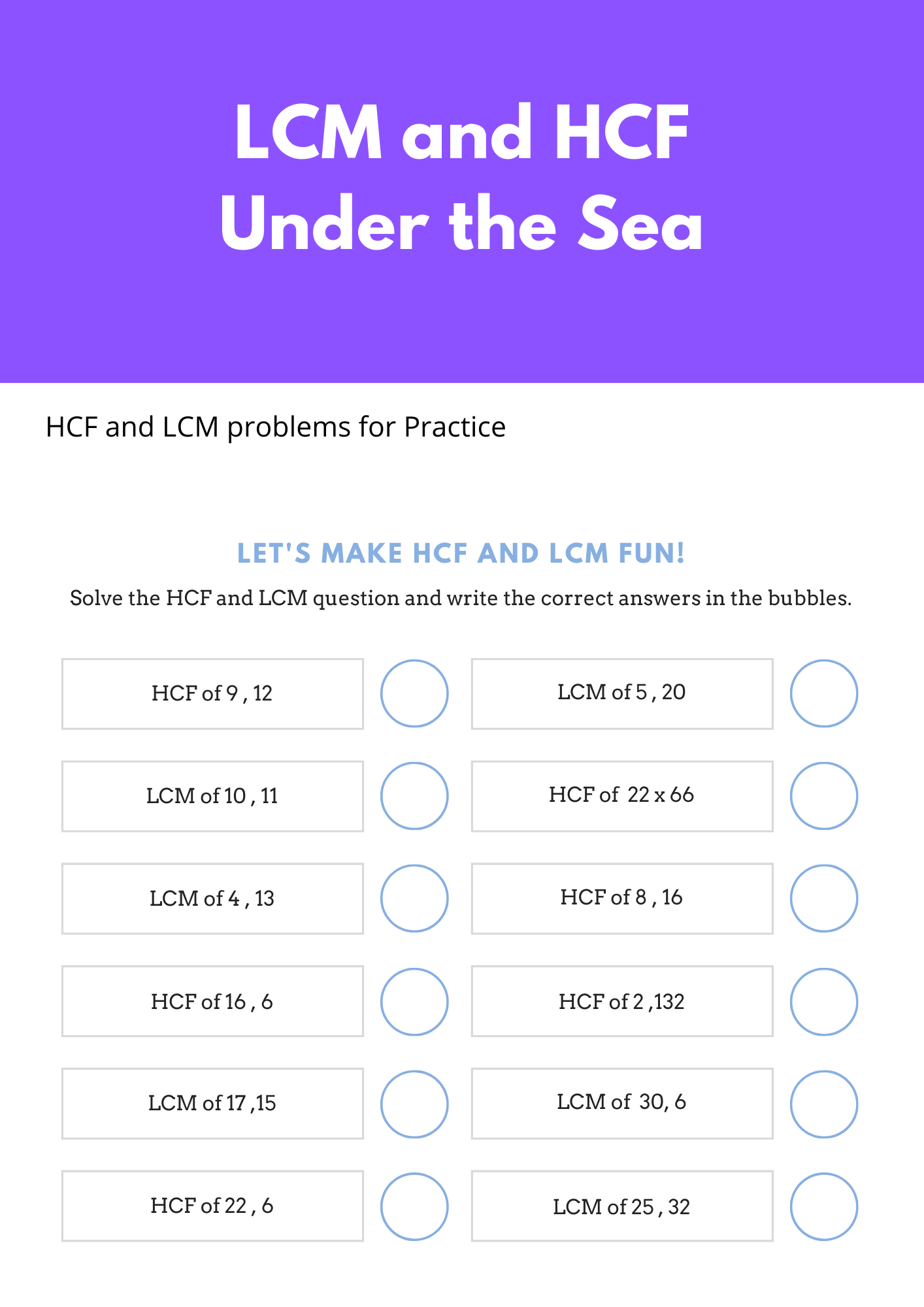
Practice HCF and LCM problems HCF and LCM questions for Class 6 Maths Playing With Numbers with Solutions

Answer

a.
$9=3 \times 3$
$12 =2 \times 2 \times 3$
HCF =3

b.
$10=2 \times 5$
$11 =1 \times 11$
LCM =110

c.
$4=2 \times 2$
$13 =1 \times 13$
LCM =52

d.
$6=2 \times 3$
$16 =2 \times 2 \times 2 \times 2$
HCF =2

e.
$17=1 \times 17$
$15 =3 \times 5$
LCM =255
f.
$6=2 \times 3$
$22 =2 \times 11$
HCF =2

g.
$5=5 \times 1$
$20 =2 \times 2 \times 5$
LCM =20

h.
$22=2 \times 11$
$66 =3 \times 2 \times 11$
HCF =22

i.
$8=2 \times 2 \times 2$
$16 =2 \times 2 \times 2 \times 2$
HCF =8

j.
$2=2$
$132 =2 \times 2 \times 3 \times 11$
HCF =2

k.
$30=2 \times 3 \times 5$
$6=2 \times 3$
LCM =30

k.
$25=5 \times 5$
$32 =2 \times 2 \times 2 \times 2 \times 2$
LCM =800