Given below are the physics numericals for class 9 sound with answers
(a) Very shorts questions
(b) shorts questions
(c) Long answer questions
(d) Table Type questions
Very Short answer
Question 1.
Define one hertz? Question 2.
Audible frequency range of a human ear is 20 hertz to 20000hertz. Express it in terms of time period? Answer
Time period for 20 Hz = 1/f= 1/20=0.05s
Time period for 20,000 Hz = 1/f=1/20000=$5 \times 10^{-5}$ s
Hence, the time period range is 0.05 s to 5 x 10-5 s.
Question 3.
Derive the relationship between wavelength, frequency and speed of sound? Answer
Speed= distance/time
or
$v= \frac {\lambda}{T}$
or
$v= \lambda \nu$
Question 4.
A tuning fork having frequency 312Hz emits a wave which has a wavelength of 1.10m. Calculate the speed of sound? Answer
$v= \lambda \nu$
$v= 1.10 \times 312=343.2$ m/s
Question 5.
A wave is moving in air with a velocity of 340m/s. Calculate the wavelength if its frequency is 512Hz?. Answer
Question 6.
A radar signal is received $2 \times 10^{-5}$ sec. After it was sent and reflected by an aeroplane. How far is the aeroplane if speed of waves is $3 \times 10^8$ m/s? Answer
Given,
Speed of wave, v = $3 \times 10^8$ m/s
Time taken for receiving the radar signal
t =$2 \times 10^{-5}$ sec
Distance travelled by the wave
= $v \times t = 3 \times 10^8 \times 2 \times 10^{-5}=6 \times 10^3$ m
In this time wave has to travel twice the distance between the aeroplane and receiver
Hence, the distance from aeroplane
= $3 \times 10^3$ m
Question 7.
A sound wave has a frequency 2 kHz and wavelength 40 cm. How long will it take to travel 1.6 km? Answer
$\nu =2000 $ hz
$\lambda = .4$ m
Speed of the sound
$v= \lambda \nu= 2000 \times .4=800$ m/s
Time taken to cover 1.6 km = 1600/800=2 sec
Question 8.
Sound waves of wavelength $\lambda$ travel from a medium in which its velocity is v m/s into another medium in which its velocity is 3 v m/s. What is the wavelength of the sound in the second medium. Frequency remains constant? Answer
$v= \lambda \nu$
$\nu= \frac {v}{\lambda}$
As frequency is constant
$\frac {v_{1}}{\lambda _{1}}=\frac {v_{2}}{\lambda _{2}}$
$\lambda _{2}= \frac {v_{2} \times \lambda _{1}}{v_{1}}= \frac { 3v \times \lambda}{v}=2 \lambda $ m
Question 9.
A person is listening to a tone of 500 Hz sitting at a distance of 450m from the source of the sound. What is the time interval between successive compressions from the source? [Speed of sound in air = 330m/s] Answer
The time interval between successive compressions from the source is equal to the time period and time period is reciprocal of the frequency. Therefore, it can be calculated as follows:
T= 1/F
T= 1/500
T = 0.002 s.
Question 10.
A boy standing in front of a wall at a distance of 85 m produces 2 claps per second. He notices that the sound of his clapping coincides with the echo. The echo is heard only once when clapping is stopped. Calculate the speed of sound.
Distance of wall from the boy=85m
To hear the echo, sound has to travel a distance =2 * 185=170 m
Since 2 claps are produced in one second, each clap is produced after =1/2=.5 sec
Since echo matches with the second clapping.
Speed of sound= 170/.5=340 m/s
Long Answer type
Question 11.
A stone is dropped from a 500 m tall building into a pond. When is sound of splash heard at the top? (g=10m/s2 , speed of sound in air= 340m/s)? Answer
Here we need to first find the time for the stone to drop in pond
$s=ut + \frac {1}{2}gt^2$
Here s=500m, u=0,g=10m/s2
$500=\frac {1}{2} \times 10 \times t^2$
or t=10 sec
Now time for the sound to travel to peak of tower
=500/340=1.47 sec
So total time to heard the sound=10 + 1.47=11.47 sec
Question 12.
Find the distance of cloud from you when you hear a thunder 3 sec after the lightning is seen. (given the speed of light= 3*108 m/s, speed of sound= 330m/s)? Why is lightning seen a few seconds before the thunder is heard during a thunderstorm? Answer
Speed of light is greater than the speed of sound. Therefore, lightning will reach in no time but the thunder will be heard 3 seconds after the lightning by the man.
We know that the lightning and the sound travels the same distance, let us consider that as 'd'.
Now for light
$d= 3 \times 10^8 t$
For sound
$d=330 \times (t+3)$ as sound is heard 3 sec later
Equating both
$3 \times 10^8 t= 330(t+3)$
Solving for t,we get
$t=3.30 \times 10^{-6}$ s
Therefore
$d=3 \times 10^8 \times 3.30 \times 10^{-6}=990$ m
Question 13.
In tank, 10 ripples are produced in one sec. If the distance between a crest and trough is 10cm, find?
(a)Wavelength
(b)Frequency
(c)Velocity of the wave? Answer
The wavelength of a wave is the distance between two consecutive crests or troughs. Here, the distance between a crest and next trough is half of the wavelength.
$\frac {\lambda}{2}=10$ cm
$\lambda$=20 cm =.2 m
Frequency is the number of oscillations per unit time.
f=10 hz
Now, velocity of the wave,
$v= \lambda \nu = .2 \times 10=2 $ m/s
Question 14.
When a wave travels from one medium to another, the wavelength changes but not the frequency. The wavelength of sound disturbance 30 cm in air and of the wave velocity is 340 m/s. What will be the wavelength of this disturbance in Helium? The speed of sound in helium is 970 m/s and 1450 m/s in water? Answer
$v= \lambda \nu$
$\nu= \frac {v}{\lambda}$
As frequency is constant
$\frac {v_{air}}{\lambda _{air}}=\frac {v_{hel}}{\lambda _{hel}}$
$\lambda _{hel}= \frac {v_{hel} \times \lambda _{air}}{v_{air}}= \frac { 970 \times .3}{340}=.85$ m
Question 15.
An engine is approaching a hill at constant speed. When it is at a distance of 0.9km, it blows a whistle, whose echo is heard by the driver after 5s. If the speed of sound is 340m/s, calculate the speed of the engine. Answer
Let v be the speed of the engine
Initial distance of engine from cliff=900m
Distance of engine from cliff when echo is heard= 900 -5v
Total distance covered by sound= 900 + 900 -5v=1800-5v
Now
Speed= distance/time
340=1800-5v/5
or
v=30 m/s
Question 16
An echo is returned in 6 seconds. What is the distance of reflecting surface from source? [Given that speed of sound is 342 m/s.] Answer
Given
Time in which echo returned, t = 6 s,
Speed of sound, v = 342 m/s
Distance = Speed × Time = 342 × 6 = 2052m
As this distance is twice the distance of reflecting surface from source.
So,
The distance of reflecting surface from source = 2052 / 2 = 1026 m.
Table Type Questions
Question 17.
Summary
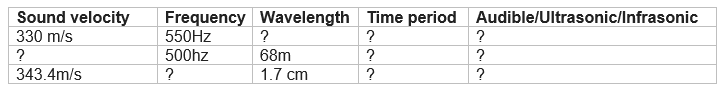
This physics numericals for class 9 sound with answers is prepared keeping in mind the latest syllabus of CBSE . This has been designed in a way to improve the academic performance of the students. If you find mistakes , please do provide the feedback on the mail.
link to this page by copying the following text Also Read
