

1. a
as $sin^{-1} x \leq \frac {\pi}{2}$
so, $sin^{-1} x=\frac {\pi}{2}$, $sin^{-1} y=\frac {\pi}{2}$,$sin^{-1} z=\frac {\pi}{2}$
or x=y=z=1
$x^{21} +y^{21} + z^{21} - 3xyz=0$
2. a
3. b
4. d
5. b
$cot (\sum_{n=1}^{23}cot^{-1}\left ( 1+\sum_{k=1}^{n} 2k\right ))= cot(\sum_{n=1}^{23}cot^{-1} ( 1+ 2\frac {n(n+1)}{2} ))$
$=cot(\sum_{n=1}^{23}cot^{-1} [ 1+ n(n+1)])= cot(\sum_{n=1}^{23}tan^{-1} \frac {1}{ 1+ n(n+1)})$
$=cot(\sum_{n=1}^{23} [ tan^{-1} (n+1) - tan^{-1} n]) =cot(tan^{-1} 24 - tan^{-1} 1) = cot tan^{-1} \frac {23}{25} =\frac {25}{23}$
6. c
7. b
$sin^{-1} \frac {x}{5} + cosec^{-1} \frac {5}{4} = \frac {\pi}{2}$
$sin^{-1} \frac {x}{5} + sin^{-1} \frac {4}{5} = \frac {\pi}{2}$
$sin^{-1} \frac {x}{5}= \frac {\pi}{2} - sin^{-1} \frac {4}{5}$
$sin^{-1} \frac {x}{5}=cos^{-1} \frac {4}{5}$
$sin^{-1} \frac {x}{5}=sin^{-1} \frac {3}{5}$
x=3
8. a
$sin^{-1} sin12 + cos^{-1} cos 12= 12 - 3 \pi + 3 \pi -12=0$
9. b
$f(x) = (sin^{-1}x)^2 + (cos^{-1}x)^2 = (sin^{-1} x + cos^{-1} x) ^2 - 2 sin^{-1} x cos^{-1} x$
$= \frac {\pi^2}{4} - 2 sin^{-1} x ( \frac {\pi}{2} - sin^{-1} x)= \frac {\pi^2}{4} - \pi sin^{-1} x +2 (sin^{-1} x)^2$
$= 2((sin^{-1} x)^2 - \frac {\pi }{2} sin^{-1} x \frac {\pi^2}{8})= 2[(sin^{-1} x - \frac {\pi}{4})^2 + \frac {\pi^2}{16}]$
So greatest and least value of the function are $\frac{5\pi ^2}{4}$, $\frac{\pi ^2}{8}$
10. b
$ sin^{-1} ( cos \frac {33\pi}{5})= sin^{-1} [ cos (6\pi + \frac {3\pi}{5})]= sin^{-1} cos( \frac {3\pi}{5}) = sin^{-1} sin(\frac {\pi}{2}- \frac {3\pi}{5}) $
$=sin^{-1} sin(\frac {-\pi}{10})=\frac {-\pi}{10} $
(i) $\frac {3\pi}{4}$
let $tan \theta =\frac {\pi}{\sqrt 2}$ or $\pi \sqrt 2 tan \theta$
So $\frac {\pi}{4} < x < \frac {\pi}{2}$
so,$\frac {3}{2} cos^{-1} \sqrt {\frac {2}{2 + \pi ^2}} +\frac {1}{4}sin^{-1} \frac {2\sqrt 2 \pi}{2 + \pi^2} + tan^{-1} \frac {\sqrt 2}{\pi}$
$=\frac {3}{2} cos^{-1} \sqrt {\frac {2}{2 + 4tan^2 \theta}} +\frac {1}{4}sin^{-1} \frac {2\sqrt 2 (2 tan \theta)}{2 + 4tan^2 \theta} + tan^{-1} cot \theta$
$=\frac {3}{2} cos^{-1} cos \theta + \frac {1}{4} sin^{-1} sin 2 \theta + tan^{-1} cot \theta$
$=\frac {3}{2} \theta + \frac {1}{4} (\pi -2 \theta) + \frac {\pi}{2} - \theta= \frac {3\pi}{4}$
(ii)$\frac {\pi}{3}$
(iii) 2
$sin^{-1}\left ( \sum_{i=1}^{\infty }x^{i+1} - x \sum_{i=1}^{\infty } (x/2)^i\right )=\frac {\pi}{2} - cos^{-1}\left ( \sum_{i=1}^{\infty }(-x/2)^{i} - \sum_{i=1}^{\infty } (-x)^i\right )$
$sin^{-1}\left ( \frac {x^2}{1-x} - \frac {x(x/2)}{1-x/2}\right )= \frac {\pi}{2} - cos^{-1}\left ( \frac {-x/2}{1+x/2} - \frac {-x}{1+x}\right )$
$sin^{-1}\left ( \frac {x^2}{(1-x)(2-x)} \right ) =\frac {\pi}{2} - cos^{-1} \left ( \frac {x}{(1+x)(2+x)}\right )$
$sin^{-1}\left ( \frac {x^2}{(1-x)(2-x)} \right ) =sin^{-1} \left ( \frac {x}{(1+x)(2+x)}\right )$
$\frac {x^2}{(1-x)(2-x)}= \frac {x}{(1+x)(2+x)}$
$x(x^3 + 2x^2 + 5x -2)=0$
so x =0 or $x^3 + 2x^2 + 5x -2=0$
Now $x^3 + 2x^2 + 5x -2=0$ is increasing function for all x
Also f(0)=-2 , f(1/2) > 0
So there lies one value in (0, 1/2) for which it will become zero
Hence total number of solutions =2
(iv) 0
$sec^{-1} \left ( \frac {1}{4} \sum_{k=0}^{10} sec \left ( \frac {7\pi}{12} + \frac {k \pi}{2} \right ) sec \left ( \frac {7\pi}{12} + \frac {(k+1)\pi}{2} \right ) \right )$
$=sec^{-1} \left ( -\frac {1}{4}\sum_{k=0}^{10} sec \left ( \frac {7\pi}{12} + \frac {k \pi}{2} \right ) cosec \left ( \frac {7\pi}{12} + \frac {k\pi}{2} \right ) \right )$
$=sec^{-1} \left ( -\frac {1}{4} \sum_{k=0}^{10} \frac {1}{sin \frac {7\pi}{6}. (-1)^k} \right )$
$=sec^{-1} \left (-\frac {1}{2} \frac {1}{sin \frac {7\pi}{6}} \right )$
$=sec^{-1} 1 =0$
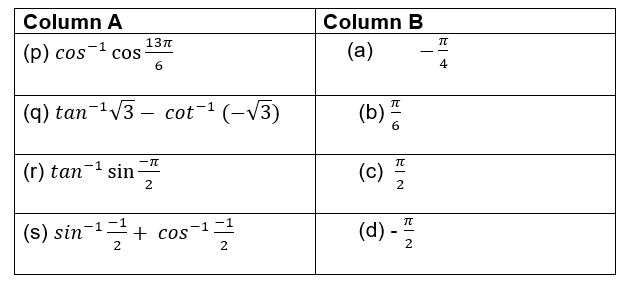
p -> b
q -> d
r -> a
s -> c