Latest Articles

combination of capacitors

Capacitors in series and parallel combinations

Q=CV

(i) Parallel combination of capacitors

Figure below shows two capacitors connected in parallel between two points A and B
Right hand side plate of capacitors would be at same common potential V_A. Similarly left hand side plates of capacitors would also be at same common potential V_B.
Thus in this case potential difference V_AB=V_A-V_B would be same for both the capacitors, and charges Q₁ and Q₂ on both the capacitors are not necessarily equal. So,
Q₁=C₁V and Q₂=C₂V
Thus charge stored is divided amongst both the capacitors in direct proportion to their capacitance.
Total charge on both the capacitors is,
     Q=Q₁+Q₂
      =V(C₁+C₂)
and
     Q/V=C₁+C₂                              (8)
So system is equivalent to a single capacitor of capacitance
     C=Q/V
where,
When capacitors are connected in parallel their resultant capacitance C is the sum of their individual capacitances.
The value of equivalent capacitance of system is greater then the greatest individual one.
If there are number of capacitors connected in parallel then their equivalent capacitance would be
C=C₁+C₂+ C₃........... (10)

(ii) Series combination of capacitors
Figure 7 below shows two capacitors connected in series combination between points A and B.
Both the points A and B are maintained at constant potential difference V_AB.
In series combination of capacitors right hand plate of first capacitor is connected to left hand plate of next capacitor and combination may be extended foe any number of capacitors.
In series combination of capacitors all the capacitors would have same charge.
Now potential difference across individual capacitors are given by
V_AR=Q/C₁
and,
V_RB=Q/C₂
Sum of V_AR and V_RB would be equal to applied potential difference V so,
V=V_AB=V_AR+V_RB
=Q(1/C₁ + 1/C₂)
or,

where

i.e., resultant capacitance of series combination C=Q/V, is the ratio of charge to total potential difference across the two capacitors connected in series.
So, from equation 12 we say that to find resultant capacitance of capacitors connected in series, we need to add reciprocals of their individual capacitances and C is always less then the smallest individual capacitance.
Result in equation 12 can be summarized for any number of capacitors i.e.,

Question 1
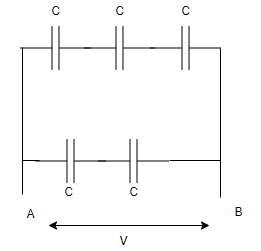
A network of five capacitors of C is connected to a 100 V supply, as shown in below figure. Determine
(a) the equivalent capacitance of the network
(b) the charge on each capacitor.

Solution
In the given network , the top three Capacitance is in series, So equivalent capacitance of top part
$\frac {1}{C_1} = \frac {1}{C}+\frac {1}{C}+ \frac {1}{C}$
$C_1 = \frac {C}{3}$
Similarly, the lower two Capacitance is in series, So equivalent capacitance of lower part
$\frac {1}{C_2} = \frac {1}{C}+\frac {1}{C}$
$C_2 = \frac {C}{2}$
Now both $C_1$ and $C_2$ are in parallel, so equivalent Capacitance of the Network
$C_{eq} = C_1 + C_2 = \frac {C}{3} + \frac {C}{2}= \frac {5C}{3}$
Now Charge on top part will be
$Q_1 = C_1 V = \frac {CV}{3}$
Now Charge on lower part will be
$Q_2 = C_2 V = \frac {CV}{2}$

Question 2
Find the equivalent Capacitance between point P and Q

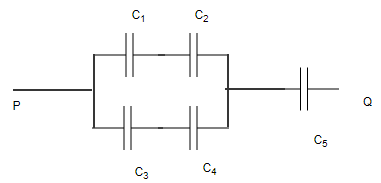
Here $C_1=2 \ \mu F$,$C_2=4 \ \mu F$,$C_3=8 \ \mu F$,$C_4=10 \ \mu F$,$C_5=12 \ \mu F$
Solution
In the given network ,$C_1$ and $C_2$ are series, so equivalent capacitance C' for that will be
$\frac {1}{C'}=\frac {1}{C_1}+ \frac {1}{C_2}$
or
$C'=4/3 \ \mu F$
In the given network ,$C_1$ and $C_2$ are series, so equivalent capacitance C'' for that will be
$\frac {1}{C''}=\frac {1}{C_3}+ \frac {1}{C_4}$
or
$C''=40/9 \ \mu F$
Now C' and C'' are in parallel,so it can be replaced with the equivalent capacitance $C_p$
$C_p=C'+ C''=52/9 \mu F$
Now $C_p$ and $C_5$ are in series, so net equivalent capacitance will be
$C_{eq} = \frac { C_p C_5}{C_p + C_5} = \frac {52/9 \times 12}{52/9 + 12} =3.9 \mu F$