Average Speed and Average Velocity

What is velocity?

Velocity is defined as the rate of change of displacement.

1. It is a vector quantity, both magnitude and direction are required to define it.
2. It's direction is same as that of displacement.
3. SI unit of velocity is m/s

What is speed?

Speed is defined as the rate of change in distance with respect to time.

1. It is a scalar quantity. Only magnitude is required to define the speed.
2. Speed and velocity both have same unit.

Average velocity

• To understand what is average velocity consider a particle moving along a straight line, as shown in figure 1, i.e. the particle is moving along the $X-axis$.
• In this case, the $X$ coordinate describing the motion of the particle from the origin $O$ varies with time, or we can say that the $X$ coordinate is time dependent.
• If at time $t=t_1$ particle is at point $P$ , at a distance $x_1$ from origin and at time $t=t_2$ it is at point $Q$ at a distance $x_2$ from the origin then displacement during this time is a vector from point $P$ to $Q$ and is
  $$\Delta x = {x_2} - {x_1} \tag{1}$$

  

• The average velocity of the particle is defined as the ratio of the displacement $\Delta x$ of the particle in the time interval $\Delta t=t_2-t_1$. If $v_{avg}$ represents average velocity then, $$v_{avg}=\frac{\Delta x}{\Delta t} \tag{2}$$



• Figure 2 represents the co-ordinate time graph of the motion of the particle i.e., it shows how the value of x-coordinate of moving particle changes with the passage of time.
• In figure 2 average velocity of the particle is represented by the slope of chord $PQ$ which is equal to the ratio of the displacement $\Delta x$ occurring in the particular time interval $\Delta t$.
• Like displacement average velocity v_avg also has magnitude as well as direction i.e., average velocity is a vector quantity.
• Average velocity of the particle can be positive as well as negative and its positive and negative value depends on the sign of displacement.
• If displacement of particle is zero its average velocity is also zero.
• Graphs below shows the x-t graphs of particle moving with positive, negative average velocity and the particle at rest.
  

  

Average Speed

• It is clear from graph (a) that for positive average velocity, the slope of the line slants upwards right, or it has a positive slope.
• The line for negative average velocity slope slants upwards and down to the right, indicating that it has a negative slope.
• For particles at rest slope is zero.
• So far we have learned that Average speed is defined as total distance travelled divided by time taken.
• Displacement of the object is different from the actual distance travelled by the particle.
• For actual distance travelled by the particle its average speed is defined as the total distance travelled by the particle in the time interval during which the motion takes place.
• Mathematically, $$\text{Average Speed}=\frac{\text{Total Distance Travelled}}{\text{Total Time}}$$
• Since distance travelled by an particle does not involve direction so speed of the particle depending on distance travelled does not involve direction and hence is a scalar quantity and is always positive.
• Magnitude of average speed may differ from average velocity because motion in case of average speed involve distance which may be greater than magnitude of displacement.
  

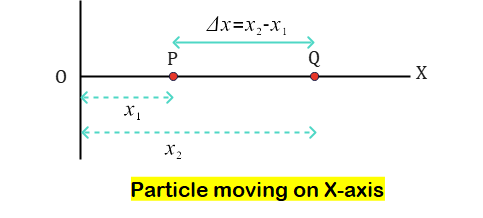
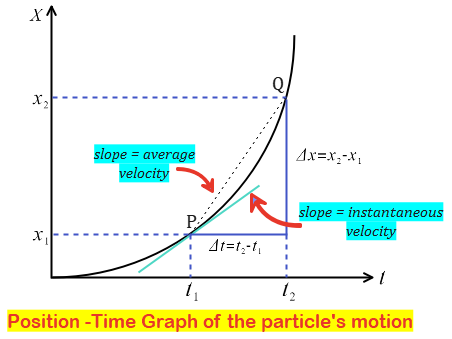
  Let us consider an example

  

  
  here a man starts travelling from origin till point $Q$ and return to point $P$ then in this case displacement of man is
  Displacement from $O$ to $Q$ is $OQ=80m$
  Displacement from $Q$ to $P$ is $=20m-80m=-60m$
  total displacement of particle in moving from $O$ to $Q$ and then moving $Q$ to $P$ is $= 80m + (-60m) = 20 m$
  Now total distance travelled by man is $OQ+OP= 80m +60m = 140m$
  Hence during same course of motion distance travelled is greater then displacement.

• From this we can say that average speed depending on distance is in general greater than magnitude of velocity.

Is average speed = average velocity?

The answer is no, average speed is not equal to average velocity. This is because
Average Speed = Total distance/Total time
Average Velocity = Total displacement/Total time
Just like both displacement and distance are different, average speed and average velocity are also different for ex. Motion around a circular track.

Average Velocity vs Average Speed

The average speed of an object is the ratio of the total distance traveled to the total time taken. The average velocity, on the other hand, is the change in position or displacement $(\Delta x)$ divided by the time intervals $(\Delta t)$ during which the displacement takes place.

Average Speed and Average Velocity Problems With Solutions

Let us now consider some of the solved examples for the concepts Average speed and Average velocity. Questions are important for understanding the concept. Try to understand these solved examples and then try to solve few questions on your own.

Examples based on Average Speed

Question 1 A car travels first half distance between two places with a speed of 40 Km/hr and the rest half with a speed of 60 Km/hr. Find the average speed of the car.
Solution Let $x$ be the total distance travelled by the car.
Time taken to travel first half distance ${t_1} = \frac{{x/2}}{{40}} = \frac{x}{{80}}hr$
Time taken to travel rest half distance ${t_2} = \frac{{x/2}}{{60}} = \frac{x}{{120}}hr$
Therefore Average speed = (Total distance)/(Total time) = $\frac{x}{{(x/80) + (x/120)}} = 48Km/hr$

Question 2 A point travelling along a straight line traverse one third the distance with a velocity $v_0$. The remaining part of the distance was covered with velocity $v_1$ for half the time and with velocity $v_2$ for the other half of the time. Find the mean velocity of the point averaged over the whole time of motion.
Solution Let $s$ be the total distance. Let $\frac{s}{3}$ be the distance covered in time $t_1$ while the remaining distance $\frac{2s}{3}$ in time $t_2$ second
$\frac{s}{3} = {v_0}{t_1}$
${t_1} = \frac{s}{{3{v_0}}}$             (1)
and,
$\frac{{2s}}{3} = {v_1}\left( {\frac{{{t_2}}}{2}} \right) + {v_2}\left( {\frac{{{t_2}}}{2}} \right)$
or,
${t_2} = \frac{{4s}}{{3({v_1} + {v_2})}}$            (2)
Average Velocity $ = \frac{s}{{{t_2} + {t_1}}}$
or, Average Velocity $ = \frac{s}{{\left( {\frac{s}{{3{v_0}}}} \right) + \left( {\frac{{4s}}{{3({v_1} + {v_2})}}} \right)}} = \frac{{3{v_0}({v_1} + {v_2})}}{{{v_1} + {v_2} + 4{v_0}}}$

Examples based on Average Velocity

Question 1 Usually "average speed" means the ratio of total distance covered to total the total time elapsed. However sometimes the phrase "average speed" can mean magnitude of average velocity. Are the two same?
Solution No, usually they have different meanings, as according to their definitions
Average speed is defined as total distance travelled divided by time taken and Average velocity is defined as change of displacement divided by the time taken. Now since distance travelled by any particle is either grater then the displacement or it is equal to the displacement , the velocity would be given as
${v_{av}} \geq |{{\vec v}_{av}}|$
that is usually average speed is greater than the magnitude of average velocity.
For example if a body returns to its starting point after some motion, then as distance travel would be finite while displacement would be zero. So in this case average speed would be greater then zero but magnitude of average velocity would be equal to zero.
However in case of motion along the straight line without change in direction, magnitude of displacement would be equal to distance and two definitions would mean the same.

Question 2 A student argues that the mean velocity during an interval of time can also be expressed as $\vec v = \frac{{{{\vec v}_f} + {{\vec v}_i}}}{2}$ and this should always be equal to $\vec v = \frac{{{{\vec r}_f} - {{\vec r}_i}}}{{{t_2} - {t_1}}}$ . is he right?
Solution: No, he is not right. The correct definition of average velocity is the later one.The first one can be used only when there is uniform acceleration.

---

Also Read

---

• Notes
  • distance and displacement
  • Velocity and speed
  • Instantaneous velocity and speed
  • Acceleration
  • Kinematic equations for uniformly accelerated Motion
  • Motion in straight line with variable acceleration with Problem and solutions
  • Free fall acceleration
  • Relative velocity
  • 1D Kinematics Problems And Solutions
  • One dimensional motion problems with solution
  • Motion graphs worksheet with Answer
• Assignments and worksheets
  • Important Questions on Kinematics
  • Kinematics worksheet
  • Motion in one dimension Practice Paper
  • Acceleration worksheet with answers
• NCERT Solutions
  • Motion in a straight line NCERT solutions

---

---

Latest Updates Sound Class 8 Science Quiz

---

Limits Class 11 MCQ

---

Circles in Conic Sections Class 11 MCQ

---

Plant Kingdom free NEET mock tests

---

The Living World free NEET mock tests

---